21 3 Blackjack Payouts
Learn how to play 21 plus 3 with this in-depth video. This covers the basics, placing a wager, playing the side bet, continue playing blackjack, and poker ra. The total blackjack payout, then, partially depends on your wager. 3:2 is the payoff for a blackjack. To win the blackjack payout of 3:2, your hand must consist an Ace and a face-card or a ten card. Facecards have a value of 10 in blackjack Such card combination is referred to as natural 21 or blackjack. This means that if you have placed a hundred-dollar bet, you will be paid $150. If you and the dealer both have a blackjack.
Blackjack is one of the most popular casino games mainly due to it being relatively easy to understand and play along with the fact that is has a very low house edge when played with optimum blackjack strategy.
As well as being able to bet on your hand against the dealers, many blackjack tables give you the option of betting on a range of side bets. There are a number of different side bets available depending on the casino you’re playing at and the individual table. Some of these side bets can return huge profits when hit for a relatively low stake on them which makes them attractive to many players. Especially when they see other players at the table winning on their side bets.
However, are side bets worth it? Do they have value? What are the odds of a side bet on blackjack?
We’ll be answering all these questions in this article to ensure you get the most value from your bets.
What Are Blackjack Side Bets?
As mentioned, there are a number of different side bets available and the ones you’ll be able to bet on will depend on the casino and table you’re playing at and if they offer them. However, there are some more common blackjack side bets which are the ones we will be focusing on in this article. Those side bets are:
- Perfect Pairs
- 21+3
- Insurance
21 3 Blackjack Payouts Table
We’ll be taking a look at these two side bets individually and showing you how they work along with a bit of math to determine whether or not they have value.
Perfect Pairs
First up we have Perfect Pairs which is perhaps the most popular blackjack side bet.
When betting on Perfect Pairs, you are betting on the two cards which you are initially dealt being the same. They don’t have to be of the same suit but the payouts are greater if they are.
For example, if you bet on Perfect Pairs and you were dealt two sixes, your side bet would win. If you were dealt two Jacks, your side bet would win, and so on.
It’s a simple side bet to understand which is why it is so popular.
What Are The Payouts For Perfect Pairs?
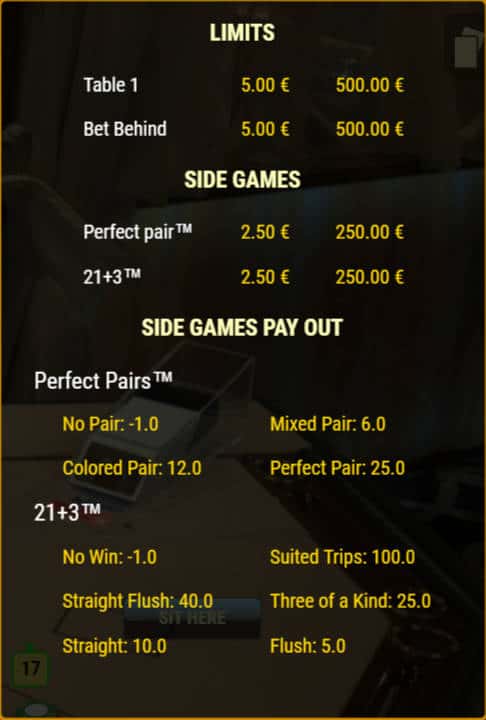
The payouts for perfect pairs vary depending on the number of decks of cards in the shoe along with the table you’re playing at. Some casinos offer different payouts on Perfect Pairs but in our example, we’ll be using the payouts which the majority of online casinos in the UK use. Typically, online casinos such as William Hill, 888Casino, Paddy Power and others use 8 decks in their shoes.
So, if you were to place a £1 Perfect Pair side bet and you were dealt 2 x 6 of hearts, your returns would be £26. This doesn’t include any potential winnings from your hand against the dealers. Regardless of whether your hand wins, you will still be paid for your winning side bet.
What Are The Odds For Perfect Pairs?
In order to decide whether or not the Perfect Pairs side bet has value, we must determine the probability of winning the side bet and compare it to the payouts. This will give us the house edge.
Perfect Pair Probability
A perfect pair, which is a pair of the same cards and the same suit, pays out at 25/1.
In a show which has 8 decks, there are a total of 416 cards. One of these cards will have already been dealt to you and so by using the number of remaining cards we can determine the probability of the same card being dealt next.
So, one card has been dealt which leaves 415 cards. Only 7 of these cards are the same as the one which you have been dealt and so the odds of you receiving a second card which is the same as your first card are 7/415 which is around a 1.69% chance.
Perfect Pair Probability = 1.69%
Coloured Pair Probability
If you receive a pair of the same cards which are of the same colour but have different suits, the payout on your perfect pair side bet is 12/1.
Again, as you have been dealt one card, there are 415 remaining cards and this time there are 8 cards remaining in the deck which could give you a coloured pair.
Therefore, there is an 8/415 chance that you will be dealt a coloured pair which is around a 1.93% chance.
Coloured Pair Probability = 1.93%
Mixed Pair Probability
The final Perfect Pair side bet which you can win is a mixed pair (one red & one black) which pays out at 6/1.
When you are dealt your first card, there are 415 cards left in the shoe and there are 16 cards remaining which will result in a coloured pair.
This means that there is a 16/416 chance that you will be dealt a coloured pair which is around a 3.86% chance.
Mixed Pair Probability = 3.86%
Perfect Pair Expected Value
Now that we’ve determined the probability of each of the perfect pair possibilities occurring, we can use the payouts offered to determine the Expected Value of each.
To do this, we simply multiply the probability by the payout odds.
Perfect Pair Expected Value = 25 x 1.69% = 0.4225
Coloured Pair Expected Value = 12 x 1.93% = 0.2316
Mixed Pair Expected Value = 6 x 3.86% = 0.2316
Perfect Pair RTP (Return To Player)
To determine the RTP of a Perfect Pair side bet, we simply need to add the three possibilities listed above together.
Perfect Pair RTP = 0.4225 + 0.2316 + 0.2316 = 0.8857 = 89%
Perfect Pair House Edge
The house edge of a Perfect Pair side bet can be determined by subtracting the RTP from 100%.
Perfect Pair House Edge = 100% – 89% = 11%
Advantages of Perfect Pairs
A house edge of around 11% is extremely high. So, why do players bet on perfect pairs if they know that the odds are stacked heavily in favour of the casino?
The main appeal to betting on perfect pairs is the potential for very high returns. Hitting a perfect pair at 25/1 early on in a session can give your bankroll a big boost.
However, it’s important to look at the bigger picture when playing any casino game and you always want to do whatever you can to keep the house edge to a minimum if the aim is to make money.
Therefore, we would advise against placing Perfect Pair side bets.
Disadvantages of Perfect Pairs
The disadvantage of Perfect Pair side bets is that they have a high house edge. If the house edge is greater than that of playing a standard hand of blackjack, then your money is better spent on the hand rather than the side bet.
21+3
21+3 is another popular side bet when playing blackjack and unlike Perfect Pairs from which you can win in 3 ways, 21+3 has 5 different ways to win.
Another difference between 21+3 and Perfect Pairs is that instead of just using the two cards which you are dealt, with 21+3, you are using your two cards as well as the dealers up-turned card.
When placing a 21+3 side bet, you are betting on any of the following occurring.
- Suited Three of a Kind: Your two cards and the dealer’s up-turned card are identical, including value, suit and colour.
- Straight Flush: Your two cards and the dealer’s up-turned card are suited and make a numerical sequence eg 6 diamonds, 7 diamonds, 8 diamonds.
- Three of a Kind: Three cards the same but not matching suits
- Straight: Three cards which make a numerical sequence but not matching suits
- Flush: Three cards of the same suit
What Are The Payouts For 21+3 Side Bets?
Just like with the Perfect Pairs side bet, payouts can vary depending on the online casino you’re playing at, the variation of the blackjack game and the number of decks being used.
The following payouts are most common for UK players playing on live dealer tables powered by providers such as Evolution Gaming.
For example, if you placed a £1 21+3 side bet and you were dealt 2 x 9 of hearts and the dealer was also dealt a 9 of hearts, you would receive £101 back along with any winnings from your hand.
21+3 Side Bets House Edge
The original 21+3 side bet paid out at 9/1 for a winning hand which resulted in a house edge of 3.24%. However, newer pay tables mean that there is greater volatility in house edges which depend on the card which you are dealt and the cards which remain in the shoe at the time of the hand being dealt.
There are different variations of 21+3 which have a house edge ranging from 2.78% all the way up to 13.39% but the theoretical RTP of a 21+3 side bet is 95.38% which gives a house edge of 4.62%.
Blackjack Insurance
The final blackjack side bet we will be covering in this article is Blackjack Insurance. Players are only offered this bet when the dealer is dealt an Ace.
Players are offered insurance after their cards have been dealt and only if the dealers up-card is an Ace.
Players can choose to take insurance for a cost of half of their original bet. Should the dealer have Blackjack, the players insurance bet will pay out at 2/1.
For example, if you placed a £10 bet on your hand too insurance after the dealer showed an Ace, the cost for the insurance bet would be £5. Should the dealer have Blackjack, you would receive back £15 from your insurance bet as well as anything you win from your hand.
Many players see insurance as a good bet as they can receive their overall stake back from the hand should the dealer have blackjack. However, in the majority of cases, it is mathematically a bad bet.
Blackjack Insurance House Edge
To make the calculations a bit easier, in this example, we will be using one 52 card deck.
If you were dealt two cards, let’s say a 6 and a 7, and the dealer was dealt an Ace, there are 49 cards remaining in the deck. 16 of these remaining cards have a value of 10 which would give the dealer Blackjack.
To determine the odds of the dealer having blackjack, we simply need to divide the number of cards in the deck which wouldn’t result in Blackjack by the number of cards which would.
Blackjack Insurance Odds = 33 / 16 = 2.0625 to 1
However, when you place an insurance bet, you only get offered odds of 2/1.
This works out as a house edge of around 2% – 3% which may not seem too bad. However, the house edge of Blackjack Insurance can fluctuate and be a lot higher.
Should your hand contain a 10-value card, then there is a reduced chance that the dealer has blackjack as there are fewer cards to do so. This increases the house edge significantly and if you are holding two 10-value cards, it can be as high as 14%.
Is Insurance A Bad Bet In Blackjack?
Based on the house edge we’ve determined above, this would make taking insurance a bad bet. However, there may be exceptions.
If you know that a lot of 10-value cards remain in the shoe, then taking insurance could be a wise bet. You will only be able to determine this if you are card counting but it can reduce the house edge significantly and in some circumstances, actually give you the edge!
- Appendices
- Miscellaneous
- External Links
Introduction
TriLux is a blackjack side bet based on the first two player cards and the dealer up card. It is similar to the 21+3 side bet, except adds a fixed bonus for the dealer on any winning hand.Rules
- The side bet is based on the use of ordinary 52-card decks.
- Winning hands are based on three cards — the initial two player cards and the dealer up card.
- There are three tables, as shown below. They show the pay table for the player, which is based on the bet amount, as usual. They also show the 'Lucky George,' which is a flat win paid to the dealer, regardless of the bet amount.
You may be wondering why the award to the dealer is called a 'Lucky George bonus.' All I know for sure is that a good tipper is called a 'George.' Meanwhile, a stingy tipper is called a 'stiff.' One theory is that a dollar bill features George Washington, and at the time that the saying caught on $1 was still a good tip.
Following are three known pay tables and Lucky George bonuses.
Pay Table 1
| Event | Player Wins | Lucky George |
|---|---|---|
| Straight flush | 8 to 1 | $1 |
| Three of a kind | 8 to 1 | $1 |
| Straight | 8 to 1 | $1 |
| Flush | 8 to 1 | $1 |
Pay Table 2
| Event | Player Wins | Lucky George |
|---|---|---|
| Straight flush | 25 to 1 | $10 |
| Three of a kind | 15 to 1 | $5 |
| Straight | 8 to 1 | $2 |
| Flush | 5 to 1 | $1 |
Pay Table 3
| Event | Player Wins | Lucky George |
|---|---|---|
| Straight flush | 25 to 1 | $10 |
| Three of a kind | 15 to 1 | $5 |
| Straight | 10 to 1 | $2 |
| Flush | 5 to 1 | $1 |
Analysis — Pay Table 1
Following is my analysis of the player's expected return of pay table 1 and six decks. The lower right cell shows a house advantage of 12.91%.
Pay Table 1 — Player Analysis
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Straight flush | 8 | 10,368 | 0.002068 | 0.016545 |
| Three of a kind | 8 | 26,312 | 0.005248 | 0.041987 |
| Straight | 8 | 155,520 | 0.031021 | 0.248171 |
| Flush | 8 | 292,896 | 0.058424 | 0.467388 |
| Loser | -1 | 4,528,224 | 0.903239 | -0.903239 |
| Total | 5,013,320 | 1.000000 | -0.129147 |
Following is my analysis of the Lucky George bonus of pay table 1 and six decks. The lower right cell shows the dealer can expect to get ¢9.68 for every hand played.
Pay Table 1 — Lucky George Analysis
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Straight flush | $1 | 10,368 | 0.002068 | $0.002068 |
| Three of a kind | $1 | 26,312 | 0.005248 | $0.005248 |
| Straight | $1 | 155,520 | 0.031021 | $0.031021 |
| Flush | $1 | 292,896 | 0.058424 | $0.058424 |
| Total | 5,013,320 | 1.000000 | $0.096761 |
The next table shows the expected return for both the player and dealer by number of decks under pay table 1.
Blackjack +3 Game
Pay Table 1 — House Edge
| Decks | Player Return | Dealer Return |
|---|---|---|
| 2 | -0.165356 | $0.092738 |
| 4 | -0.138195 | $0.095756 |
| 5 | -0.132766 | $0.096359 |
| 6 | -0.129147 | $0.096761 |
| 8 | -0.124624 | $0.097264 |
Analysis — Pay Table 2
Following is my analysis of the player's expected return of pay table 2 and six decks of cards. The lower right cell shows a house advantage of 23.25%.
Pay Table 2 — Player Analysis — Six Decks
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Straight flush | 25 | 10,368 | 0.002068 | 0.051702 |
| Three of a kind | 15 | 26,312 | 0.005248 | 0.078726 |
| Straight | 8 | 155,520 | 0.031021 | 0.248171 |
| Flush | 5 | 292,896 | 0.058424 | 0.292118 |
| Loser | -1 | 4,528,224 | 0.903239 | -0.903239 |
| Total | 5,013,320 | 1.000000 | -0.232521 |
Following is my analysis of the Lucky George bonus of pay table 2 with six decks. The lower right cell shows the dealer can expect to get ¢16.74 for every hand played.
Pay Table 2 — Lucky George Analysis — Six Decks
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Straight flush | $10 | 10,368 | 0.002068 | $0.020681 |
| Three of a kind | $5 | 26,312 | 0.005248 | $0.026242 |
| Straight | $2 | 155,520 | 0.031021 | $0.062043 |
| Flush | $1 | 292,896 | 0.058424 | $0.058424 |
| Total | 5,013,320 | 1.000000 | $0.167389 |
The next table shows the expected return for both the player and dealer by number of decks under pay table 2.
Pay Table 2 — House Edge
| Decks | Player Return | Dealer Return |
|---|---|---|
| 2 | -0.266529 | $0.159338 |
| 4 | -0.241138 | $0.165330 |
| 5 | -0.235977 | $0.166562 |
| 6 | -0.232521 | $0.167389 |
| 8 | -0.228185 | $0.168430 |
Analysis — Pay Table 3
Following is my analysis of the player's expected return of pay table 3. The lower right cell shows a house advantage of 17.05%.
Pay Table 3 — Player Analysis — Six Decks
21 3 Blackjack Payouts Free
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Straight flush | 25 | 10,368 | 0.002068 | 0.051702 |
| Three of a kind | 15 | 26,312 | 0.005248 | 0.078726 |
| Straight | 10 | 155,520 | 0.031021 | 0.310214 |
| Flush | 5 | 292,896 | 0.058424 | 0.292118 |
| Loser | -1 | 4,528,224 | 0.903239 | -0.903239 |
| Total | 5,013,320 | 1.000000 | -0.170479 |
Following is my analysis of the Lucky George bonus of pay table 3 and six decks. The lower right cell shows the dealer can expect to get ¢16.74 for every hand played.
Pay Table 3 — Lucky George Analysis — Six Decks
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Straight flush | $10 | 10,368 | 0.002068 | $0.020681 |
| Three of a kind | $5 | 26,312 | 0.005248 | $0.026242 |
| Straight | $2 | 155,520 | 0.031021 | $0.062043 |
| Flush | $1 | 292,896 | 0.058424 | $0.058424 |
| Total | 5,013,320 | 1.000000 | $0.167389 |
The next table shows the expected return for both the player and dealer by number of decks under pay table 3.
21 3 Blackjack Payouts Today
Pay Table 3 — House Edge
| Decks | Player Return | Dealer Return |
|---|---|---|
| 2 | -0.203268 | $0.159338 |
| 4 | -0.178794 | $0.165330 |
| 5 | -0.173814 | $0.166562 |
| 6 | -0.170479 | $0.167389 |
| 8 | -0.166292 | $0.168430 |
21 Blackjack Free Game
Combined Casino Cost
Although this site usually looks at games from the player's perspective, I will break from that with the following tables. They show the expected profit according to the amount of the player's bet and the pay table for six and two decks. These figures are lower than the house edge figures in the tables above because the casino must pay the player winnings and the Lucky George bonus.
Blackjack 21+3 Top 3
House Edge from Casino Perspective — Six Decks
| Bet | Pay Table 1 | Pay Table 2 | Pay Table 3 |
|---|---|---|---|
| $100 | 12.82% | 23.08% | 16.88% |
| $50 | 12.72% | 22.92% | 16.71% |
| $25 | 12.53% | 22.58% | 16.38% |
| $20 | 12.43% | 22.42% | 16.21% |
| $15 | 12.27% | 22.14% | 15.93% |
| $10 | 11.95% | 21.58% | 15.37% |
| $5 | 10.98% | 19.90% | 13.70% |
| $3 | 9.69% | 17.67% | 11.47% |
| $2 | 8.08% | 14.88% | 8.68% |
| $1 | 3.24% | 6.51% | 0.31% |
House Edge from Casino Perspective — Two Decks
| Bet | Pay Table 1 | Pay Table 2 | Pay Table 3 |
|---|---|---|---|
| $100 | 16.44% | 26.49% | 20.17% |
| $50 | 16.35% | 26.33% | 20.01% |
| $25 | 16.16% | 26.02% | 19.69% |
| $20 | 16.07% | 25.86% | 19.53% |
| $15 | 15.92% | 25.59% | 19.26% |
| $10 | 15.61% | 25.06% | 18.73% |
| $5 | 14.68% | 23.47% | 17.14% |
| $3 | 13.44% | 21.34% | 15.02% |
| $2 | 11.90% | 18.69% | 12.36% |
| $1 | 7.26% | 10.72% | 4.39% |
External Links
21 3 Blackjack Payouts List
- Wizard of Vegas — Discussion of this side bet.
Written by: Michael Shackleford